Teaching Senior School Calculus and Statistics
This package contains pdf book (over 400 pages) with supporting video (over 22 hours with about 158 video clips) that unpacks the logic behind most of the calculus (logarithms; trigonometry; functions including trig functions; exponential functions; their derivatives and integration to the volumes of rotating solids) and statistics (to hypothesis testing with Z and t tests) and binomial probability.
Designed for:
Teachers of senior school mathematics and students who seek a deeper conceptual understanding of the rules associated with calculus, binomial probability and statistics. (complete eBook with numerous worked examples).
-
SM_0 # Introduction to Teaching Senior School Calculus and Statistics Package
-
SM_1-1 # Introduction to Index
-
SM_1-2 # Index - Introducing the Concept
-
SM_1-3 # Exponential Application
-
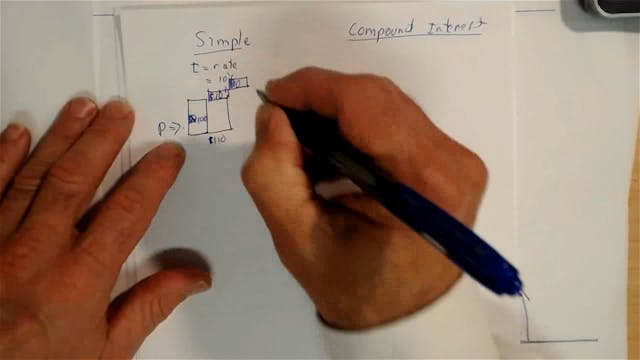
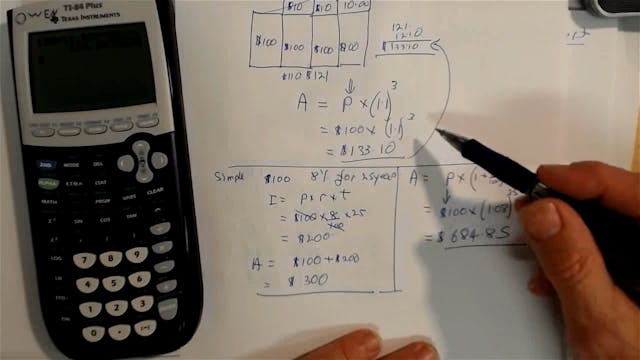
SM_1-4 # Exponentials - Simple and Compound Interest compared
-
SM_1-5 # Exponentials - Compound Interest
-
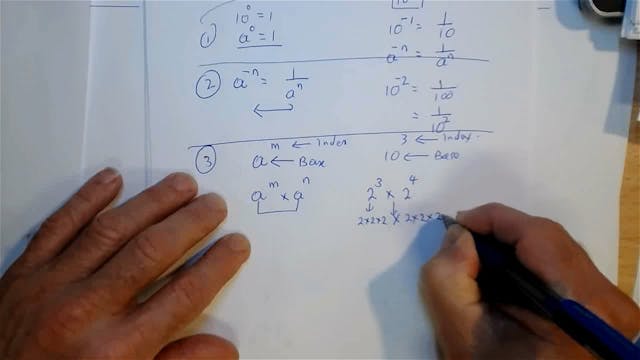
SM_1-6 # Developing Index Rules - Part A
-
SM_1-7 # Developing Index Rules - Part B
-
SM_1-8 # Index - Practice A
-
SM_1-9 # Index - Simplify (Hard) - Practice B
-
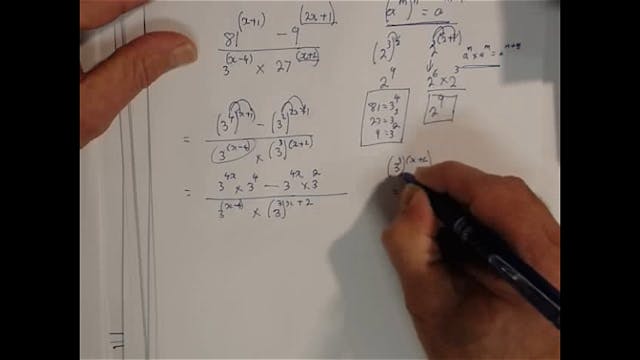
SM_1-10 # Solving in Index Contexts (Simple)
-
SM_1-11 # Solving in Index Contexts (Hard)
-
SM_1-12 # Introduction to Surds and congugate Surds
-
SM_1-13 # Surds - Simplification Practice
-
SM_1-14 # Introduction to Logarithms
-
SM_1-15 # Log Rules - Proofs and Logic
-
SM_1-16 # Log Laws - Manipulation Practice
-
SM_1-17 # Graphing Exponentials - by Hand
-
SM_1-18 # Graphing Exponentials - exploring with Computer
-
SM_1-19 # Natural Logs - Definition and Solving
-
SM_1-20 # Problem Solving in Finance with Logarithms
-
SM_1-21 # Population Problem using Index Rules
-
SM_1-22 # Population Problem using Natural Logarithms
-
SM_1-23 # Logarithms and Sound problem solving
-
SM_1-24 # Introduction to Log Graphs - Corona Virus
-
SM_1-25 # Log Problem - Virus Growth Factor
-
SM_1-26 # Virus Problem - Doubling Time
-
SM_1-27 # Introduction to Change of Base for Logs
-
SM_1-28 # Deriving the Change of Base Rule
-
SM_2-1 # Trigonometry - Introduction and Overview
-
SM_2-2 # Triangles - Basic, Pythagoras derived
-
SM_2-3 # Triangles - Congruent, Similar
-
SM_2-4 # Properties of Circles
-
SM_2-5 # Sine Ratio
-
SM_2-6 # Exact Values in Sine, Cosine, Tangent
-
SM_2-7 # Signs around the circles for Sine, Cosine, Tangent
-
SM_2-8 # Worked Example Solution ASTC
-
SM_2-9 # Introduction to Radians
-
SM_2-10 # Radians to Degrees Conversions
-
SM_2-11 # Radians to Degrees Calculators
-
SM_2-12 # Plotting Periodic Functions - Physical to Graph
-
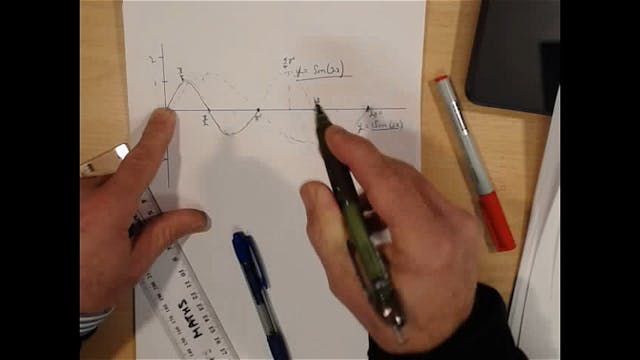
SM_2-13 # Graphing Sine Functions - Manual Coordinates
-
SM_2-14 # Graphing Cosine Functions
-
SM_2-15 # Using Technology to graph Sine Functions
-
SM_2-16 # Sine and negative Sine explained
-
SM_2-17 # Cosine and negative Cosine explained
-
SM_2-18 # Teaching the Plotting of Tangent Functions
-
SM_2-19 # Features of a Tangent Function on the Computer
-
SM_2-20 # Deriving the Equation of a Circle
-
SM_2-21 # Formalising trigonomic Relationships
-
SM_2-22 # Rearranging trigonomic Relationships with Algebra
-
SM_2-23 # Deriving and Using the Sine Rule
-
SM_2-24 # Deriving the Cosine Rule
-
SM_2-25 # Problem Solving with Cosine, Summary
-
SM_3-1 # Introduction to Probability and Binomial Expansion
-
SM_3-2 # Pascal Numbers - continued
-
SM_3-3 # Developing Binomial Generality
-
SM_3-4 # Applying Binomial Probability and Calculators
-
SM_3-5 # Binomial Problem - with Calculator
-
SM_3-6 # Binomial Expansion in Algebra
-
SM_3-7 # Introduction to Expected Value
-
SM_3-8 # Expected Value Example, Concluding Thoughts
-
SM_4-1 # Introduction to Statistics
-
SM_4-2 # Definition of Variables
-
SM_4-3 # Introduction to Sampling Methods
-
SM_4-4 # Mode, Median, Mean
-
SM_4-5 # Introduction to Box and Whisker Plots
-
SM_4-6 # Introduction to Normal Distribution, Measuring Spreads
-
SM_4-7 # Normal and Skew
-
SM_4-8 # Z Scores
-
SM_4-9 # Overview of Hypothesis Testing
-
SM_4-10 # Critical Values for Normal Distribution
-
SM_4-11 # Impact of Sample Size on Z Scores
-
SM_4-12 # Critical Values - 2 Tails and 1 Tail Tests
-
SM_4-13 # Dependent and Independent Samples
-
SM_4-14 # Using Z Scores to Calculate Difference between Means
-
SM_4-15 # Worked Examples of Z-Tests
-
SM_4-16 # When to use a T-Test
-
SM_4-17 # Comparing the Means of two Populations with T-Test
-
SM_4-18 # Using Technology to compare Means with T-Test
-
SM_4-19 # T-Test the Small Sample
-
SM_4-20 # Compare Means with SPSS
-
SM_4-21 # Introduction to Correlation
-
SM_4-22 # Running a Correlation Test with SPSS
-
SM_4-23 # Regression Equations, Conclusions
-
SM_5-1 # Introduction to Calculus, Overview of Teaching Models
-
SM_5-2 # Establishing a Need for Derivative Functions
-
SM_5-3 # Connecting Gradient Change via Graphs
-
SM_5-4 # Using a Computer to understand Gradient Functions
-
SM_5-5 # Derivative of 1 on x
-
SM_5-6 # Composite Functions and their Derivatives
-
SM_5-7 # Developing the Concept of Limits
-
SM_5-8 # Formalising Derivative from First Principles
-
SM_5-9 # Derivatives from First Principles - Practice
-
SM_5-10 # Developing the Chain Rule
-
SM_5-11 # Teaching the Product Rule of Differentiation
-
SM_5-12 # Teaching the Quotient Rule of Differentiation
-
SM_5-13 # Introduction to the Second Derivative
-
SM_5-14 # Introduction to Problem Solving with Derivatives
-
SM_5-15 # Worked Example - Simple Modelling and Problem Solving
-
SM_5-16 # Worked example - More Diffiuclt, Max Area
-
SM_5-17 # Worked Example involving Volume
-
SM_5-17 # Worked Example 2
-
SM_5-18 # Worked Example simple MAP
-
SM_5-19 # Worked Example involving Volume
-
SM_6-1 # Summary of Trigonometric Identities
-
SM_6-2 # Introduction to the Derivative of the Sine Function
-
SM_6-3 # Conceptualisaing Derivatives of Periodic Functions
-
SM_6-4 # Simple Worked Examples with Derivatives of Sine and Cosine
-
SM_6-5 # Introduction to the Derivative of Tangent Functions
-
SM_6-6 # Exploring Tangent Derivatives with Graphs
-
SM_6-7 # Deriving the Derivative of Tangent with Algebra
-
SM_6-8 # Confirming the Derivative of Tan x with a Computer
-
SM_6-9 # Making Sense of the Tangent Derivatives with a Computer
-
SM_7-1 # Introduction to e and Revision of Exponential Functions
-
SM_7-2 # Derivatives of Exponentials, Finding the Constant with Algebra
-
SM_7-3 # Calculating the Constant to find the Derivative of an Exponential Function
-
SM_7-4 # Derivative of e to the x Power
-
SM_7-5 # Simple Worked Examples
-
SM_7-6 # Using Natural Logs to find the Constant for Derivatives of Exponentials
-
SM_7-7 # Chain Rule with Exponentials
-
SM_7-8 # Using Technology to investigate the Derivative of ln(x)
-
SM_7-9 # Teaching Derivatives of log(x)
-
SM_7-10 # Derivative of log(x) - other Representation
-
SM_7-11 # Simple Worked Examples of Derivative of ln(x) Functions
-
SM_7-12 # More Worked Examples of Procedure
-
SM_7-13 # Simple Modelling Problem
-
SM_8-1 # Introduction to Integration
-
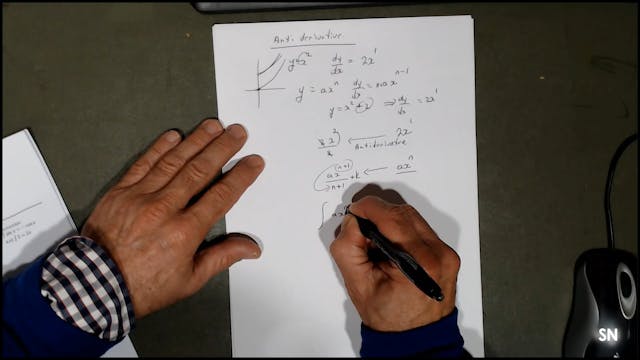
SM_8-2 # Introducing the Anti-derivative Concept
-
SM_8-3 # Connecting Differentiation and Integration
-
SM_8-4 # Linking Estimations of Areas under Curves with Formal Integration
-
SM_8-5 # Developing the Fundamental Theorem of Calculus
-
SM_8-6 # Introduction to Integration by Substitution
-
SM_8-7 # Substitution worked examples
-
SM_8-8 # Integration definite integral substitution methods
-
SM_8-9 # Simple Motion - Problem Solving with Integration
-
SM_8-10 # Introduction to the Integration of trigonomic Functions
-
SM_8-11 # Formalising the Integration of Trigonometric Functions
-
SM_8-12 # Integral of Sine linked to Fundamental Theory
-
SM_8-13 # Integral of sin(x) with Approximation Methods
-
SM_8-14 # Algebraic Approach to Deriving the Formula for Integral of sin(3x)
-
SM_8-15 # Integration of Functions 1 over x
-
SM_8-16 # Integral of Exponentials
-
SM_8-17 # Introduction to Volume of a Cone with Integration
-
SM_8-18 # Rotating Cubic Function Integration with Limits
-
SM_8-19 # Introduction to Volume of a Sphere
-
SM_8-20 # Derivation of Volume of a Sphere with Integration
-
SM_8-21 # Volume of a Solid rotated about y Axis
-
SM_8-22 # Simple Area under the Curve with a Computer
-
SM_8-23 # Introduction to Simpson's Rule of Approximation
-
SM_8-24 # Simpson's Rule with more than two Parts
-
SM_8-25 # Rotating Solids - Washer, Method 1
-
SM_8-26 # Washer, Example 2
-
SM_8-27 # Tedious Washer Problem
-
SM_8-28 # Washer, Rotated about y Axis - Simple
-
SM_9-1 # Introduction to Queensland Maths Syllabi
-
SM_9-2 # Background to Teaching Maths Methods
-
SM_9-3 # Overview of ATAR
-
Stephen Norton - Teaching Senior School Calculus and Statistics ### Study Guide
163 KB
-
Stephen Norton - Teaching Senior School Calculus and Statistics 2016.pdf
11.1 MB